Percentage Composition

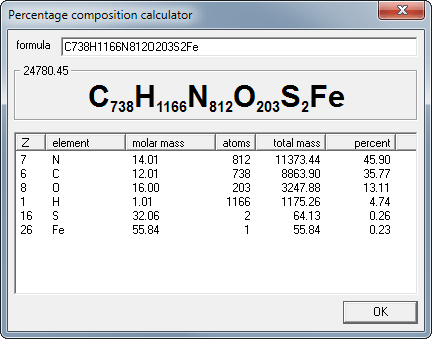
What is percentage composition?
The percent composition (percentage composition) of a compound is a relative measure of the mass of each different element present in the compound.
To calculate the percent composition (percentage composition) of a compound


Calculate the molecular mass (molecular weight, formula mass, formula weight), MM, of the compound


Calculate the total mass of each element present in the formula of the compound


Calculate the percent compositon (percentage composition): % by weight (mass) of element
= (total mass of element present ÷ molecular mass) x 100

Example 1. 
What percentage of the mass of carbon dioxide (CO2) is made up by the carbon?
Solution:
first find the mass of the total compound.
C = 12.0 u x 1 atom  = 12.0 u
O = 16.0 u x 2 atoms = 32.0 u
                                --------
                                 44.0 u
next use the formula:

                                                                                 partial mass from carbon
% of the mass of  CO2 that is made up by carbon  = -------------------------- x 100
                                                                                 total mass of the CO2

                                                                                          12.0 u
% of the mass of  CO2 that is made up by carbon  = ------------------------- x 100
                                                                                           44.0 u

% of the mass of  CO2 that is made up by carbon  = 27.3%

Answer = 27.3%
     Now, it is more typical to be asked what the percentage composition of the entire compound is.  In the example above, you can assume that if carbon makes up approximately 27% of the mass of carbon dioxide then oxygen makes up about 73%, for the total must be 100%.

Example 2. 
What is the percentage composition of glucose (C6H12O6) ?
solution:
find the mass of the entire molecule,
C = 12.0 u x 6 atoms   = 72.0 u
H = 1.01 u x 12 atoms = 12.1 u
O = 16.0 u x 6 atoms   = 96.0 u
                                ----------
                                 180 u
Then use the formula for each element in the compound:
                                                                                                  partial mass from element
% of the mass of the compound that is made up by an element = -------------------------- x 100
                                                                                                  total mass of the compound

                              72.0 u
% for Carbon = ---------------- x 100 = 40.0%                      
                              180 u

                                  12.1 u
% for Hydrogen = ---------------- x 100 = 6.7 %                        
                                  180 u

                              96.0 u
% for Oxygen = ---------------  x 100  = 53.3%                 
                              180 u
One way to check your answer is to make sure that all of the percentages add up to approximately 100%.  (i.e. 40.0% + 6.7% + 53.3% = 100%)   Your total may be off by a few tenths of a percent, due to rounding.

Example 3.
Calculate the percent by weight of each element present in ammonium phosphate [(NH4)3PO4]

Calculate the molecular mass (MM) of (NH4)3PO4:
MM = 3x[14.01 + (4 x 1.008)] + 30.97 + (4 x 16.00) = 3 x [14.01 + 4.032] + 30.97 + 64.00 = (3 x 18.042) + 30.97 + 64.00 = 54.126 + 30.97 + 64.00 = 149.096

Calculate the total mass of N present:
3 N are present, mass = 3 x 14.01 = 42.03

Calculate the percent by mass of N present in (NH4)3PO4:
%N = (mass N ÷ MM) x 100 = (42.03 ÷ 149.096) x 100 = 28.19%

Calculate the total mass of H present:
12 H are present in the formula, mass = 12 x 1.008 = 12.096

Calculate the percent by mass of H present in (NH4)3PO4:
%H = (mass H ÷ MM) x 100 = (12.096 ÷ 149.096) x 100 = 8.11%

Calculate the total mass of P present:
1 P is present in the formula, mass = 30.97

Calculate the percent by mass P in (NH4)3PO4:
%P = (mass P ÷ MM) x 100 = (30.97 ÷ 149.096) x 100 = 20.77%

Calculate the total mass of O present:
4 O are present in the formula, mass = 4 x 16.00 = 64.00

Calculate the percent by mass of O in (NH4)3PO4:
%O = (mass O ÷ MM) x 100 = (64.00 ÷ 149.096) x 100 = 42.93%

The answers above are probably correct if %N + %H + %P + %O =100, that is,
28.19 + 8.11 + 20.77 + 42.93 = 100 %




Here is a video on YouTuBe:

Mole Map

Grams ↔ Moles

Number of moles = (# of grams) ÷ (molar mass)
Number of grams = (# of moles) × (molar mass)
Example 1:How many moles are in 5 grams of O₂?
The molar mass of O₂ = 16.00 g/mole x 2 (for 2 atoms of oxygen) or 32.00 g/mole.
5 grams of O₂ ÷ (32 g/mole) = 0.15625 moles

Example 2:
How many grams does 4 moles of NH₃ weigh?
The molar mass of NH₃ = 14.01 + (3 × 1.01) = 17.04 g/mole
4 moles × 17.04 g/mole = 68.16 grams

Atoms ↔ Moles

Number of moles = (# of atoms) ÷ (6.02x10^23)
Number of atoms = (# of moles) × (6.02x10^23)

Example 1:

 1.65 × 10²⁴ atoms of Magnesium?

 1.65 × 10²⁴ atoms ÷ (6.02 × 10²³) = 2.74 moles of Mg

Moles to Gram Conversion

Gram to Mole Conversion

Mole Conversions

Above are 2 videos that show how grams are converted to moles and how moles are converted to grams.

The atomic mass is the mass of the mass of one atom of an element in ATOMIC MASS UNITS (u). This number can be found on the periodic table.
Example:
The atomic mass of Magnesium is 24.3 u. 

The formula mass is the total mass of all atoms in a covalent, organic, or polyatomic compound in ATOMIC MASS UNITS (u).
Example:
The formulas mass of NaCl is:  23.0 + 35.5 = 58.5 u.

The molecular mass is the total mass of all atoms in an ionic compound in ATOMIC MASS UNITS (u).
Example:
The molecular mass of CO is: 12.0 + 16.0 = 28.0 u.


We now know how to do conversions:
(a) from particles/atoms/formula units/molecules ----> moles
(b) from moles ----> particles/atoms/formula units/molecules
(c) from grams ----> moles
and  (d) from moles ----> grams

*With all these conversions, the molar mass is needed.
The molar mass is the same number of the molecular, atomic, or formula masses, except expressed in grams per mole (g/mole).

 

Mole day is at 6:22 on October 23 (10/23)

Equal Numbers in Equal Volumes: Avogadro

Relative Mass:
Expressed by company it mathematically to the mass of another object.

Avogardo's Law:
Equal volume of all gases under same conditions of temperature and pressure contain equal no of molecules.

Things to understand about Avogadro's number:

• It is a number, just as is "dozen", and thus is dimensionless; you can think of Avogadro's number as the "chemist's dozen".

• It is a huge number, far greater in magnitude than we can visualize;

• Its practical use is limited to counting tiny things like atoms, molecules, "formula units", electrons, or photons.

• Its value can be known only to the precision that the number of atoms in a measurable weight of a substance can be estimated. Because large numbers of atoms cannot be counted directly, a variety of ingenious indirect measurements have been made involving such things as brownian motion and X-ray scattering.

Several related terms are used to express the mass of one mole of a substance.

• Molecular weight This is analogous to atomic weight: it is the relative weight of one formula unit of the compound, based on the carbon-12 scale. The molecular weight is found by adding atomic weights of all the atoms present in the formula unit. Molecular weights, like atomic weights, are dimensionless}; i.e., they have no units.
• Formula weight The same thing as molecular weight. This term is sometimes used in connection with ionic solids and other substances in which discrete molecules do not exist.
• Molar mass The mass (in grams, kilograms, or any other unit) of one mole of particles or formula units. When expressed in grams, the molar mass is numerically the same as the molecular weight, but it must be accompanied by the mass unit.

What is the formula weight of copper(II) chloride, CuCl₂?
Answer: the atomic weights of Cu and Cl are, respectively 63.55 and 35.45;
63.55 + 2(25.35) = 134.45.

What is the molar mass of copper(II) chloride, CuCl₂?
Answer: the masses of Cu and Cl are, respectively, 63.55 g and 35.45 g;
(63.55 g) + 2(25.35 g) = 134.45 g.

Using graph to find density of water

The density of a substance is defined as the mass divided by the volume: d=m / v. Using graphing techniques, a plot of mass vs. volume will yield a slope (Δy/Δx) of density. 

Density is a physical property of a substance that does not depend on the amount of material present and is therefore called an intensive property. In this experiment, you will find the mass of water for five different volumes and plot them. Using a line of best fit, the slope will give you the value of density for water.

Always, water with different temperent has different density.

The density of water is approximately one gram per cubic centimeter. More precisely, it is dependent on its temperature, but the relation is not linear and is not even monotonic (see right-hand table). When cooled from room temperature liquid water becomes increasingly dense, just like other substances. But at approximately 4 °C, pure water reaches its maximum density. As it is cooled further, it expands to become less dense.

When the water molecule makes a physical phase change its molecules arrange themselves in distinctly different patterns (Figure 8a-2). The molecular arrangement taken by ice (the solid form of the water molecule) leads to an increase in volume and a decrease in density.

Determining Aluminum Foil Thickness Lab

Two common concepts used to measure the thickness of a small rectangular piece of aluminum foil:
1. Volume of a rectangular solid V=  Length × Width × Height
2. Density of a substance D= Mass / Volume

Steps

Calculate the volume of your aluminum piece.
Use the density formula, D = mass/volume for this. Solving for V gives
V = mass / density
(Volume of foil = ________ cubic centimeters = ______ ml)
Note: 1 cubic centimeter occupies the same volume as 1 milliliter.
Round your answer to match the origincal measurement that had the fewest significant digits.
(Volume after rounding for significant digits = _________________ ml)
Calculate the thickness of your aluminum.
Use the formula of a box, V = length x width x height
solve the formula for height, which represents the thickness of the aluminum foil in cm.
(h = _______________ cm thick)
Round your answer to match the original measurement with the fewest significant digits.
(Thickness after rounding significant digits = __________________ cm.)

Read more: http://www.brighthub.com/education/k-12/articles/8105.aspx#ixzz14NtZfEfy

Conclusion:
Two formulas provided above are important for the calculations. We should use them correctly to get an more accurate thickness.

Here is an example of the measurement of thin thickness thing:
http://ip.com/patapp/CN101349557

Egg Density Experiment

I found this experiment interesting:

DENSITY

Density= Mass/Volume

Volume=Mass/Density

Mass= (Density)(Volume)

Some common units: g/cm3 for solids and g/mL for liquids

 

As you can see from the picture on the left, the rock's density caused the volume of the water to rise. The denser the solid dropped into the liquid, the higher the volume of the liquid will be.

A material will sink if its density is greater than the density of the liquid.

A material will float if its density is less than the density of the liquid.

Try these out for further practice:

1)A block of beeswax has a volume of 200.0 mL and a density of 961 g/L. What is the mass of the block?

2)A 70.0 g of manganese (density = 7.20 x 10^3 g/L) is dropped into a graduated cylinder containing 54.0 mL of water. What will be the water level indicated after the sphere is inserted?

*Remember to start Lab 2E for next day!*